Patterning and Algebra Unit #1

Last Wednesday, we started our new unit on Patterning and Algebra. As an introduction, we looked at Fibonacci numbers and how these sequences appear in nature. The big idea is that Math is all around us, even in nature!

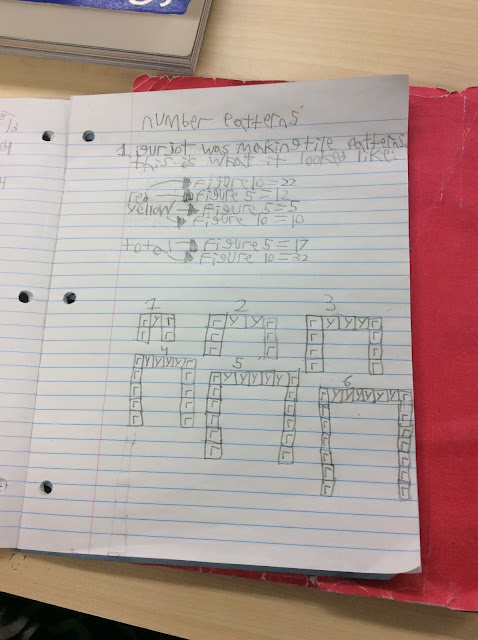
The student's first task is below:

 Some students used colour tiles to extend the pattern ....

Conclusion: If the pattern is extended to Figure 5, there will be 12 red and 5 yellow tiles for a total of 17 tiles.

In Figure 10, there will be 22 red tiles and 10 yellow tiles for a total of 32 tiles.

For homework, students had to figure out the amount of red/yellow tiles if the pattern was extended to the 100th figure as well as the total number of tiles.

Before taking up the homework, I wanted to make absolute sure that everyone in the class understood how to use a table of values in order to identify and continue a pattern.

Hence, the following task was given. I was curious to see what strategy the students would use. Would they sketch all the figures like some had done the day before or would they use a table of values? I was pleased with what was observed :-)

Task #3

Extension: How many squares will be in the 100th figure?

We realised that using algebra or shortcuts was the most efficient way to solve for the 100th term in a number pattern. Creating rules or formulas to find the unknown or variable was fun and engaging - similar to figuring out a puzzle!

We revisited our colour tile pattern question and discovered that the solution to finding the 100th term for the number of red and yellow tiles was easy once we could come up with an algebraic expression:

The same could be said of our number of squares pattern question.