Challenge - Painted Sides of a Cube

Students' Work

Not all the groups arrived at the correct answer but the strategies used were impressive. Good job to all!

Solution

a) Dimensions 1 cm x 1 cm x 1 cm

Volume 1 cm 3

b) Three red faces - 8 cubes

c) Two red faces - 24 cubes

One red face - 24 cubes

d) No red paint - 8 cubes

 Goal of today's task:
- to determine whether students can independently figure out that the task requires them to find the volume of various 3D shapes (length x width x height)
- to get students to relate, in a concrete manner, that volume is really the amount of 3D space that an object occupies
- to deepen students' understanding of the differences between a cube and a rectangular prism (cubes have all sides equal, since they have 6 faces that are squares / rectangular prisms have 4 faces that are rectangular and 2 that are squares, therefore the sides are all not equal)

Take a look of the work my kiddies came up with. Some of the calculations were off but in general it is clear from their work samples that they have a conceptual understanding of volume. Good job!

How many nets for a cube?

Review of number of edges, vertices and faces for 3D shapes. Great interactive website at:

http://interactivesites.weebly.com/geometry-shapes.html

Minds On: Visualising 

This act of visualising the folding of a net is one of the essential reasons for working with them, and is far more important mathematically than simply constructing solids from their nets.

Main task: Students were not shown the sketches below while they worked on the task. They had to independently figure out the number of nets by using Polydrons to make possible nets for a cube. Some students were convinced there were 10, 11 or 12 possible nets. The correct answer? There are 11 nets for a cube.

Faces, Vertices and Edges of Pyramids and Prisms

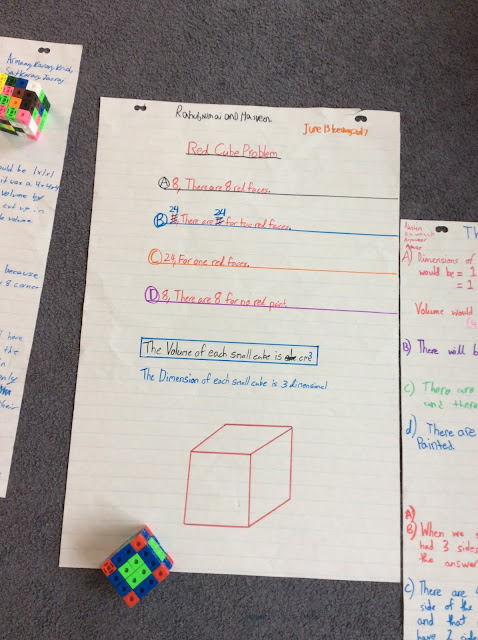
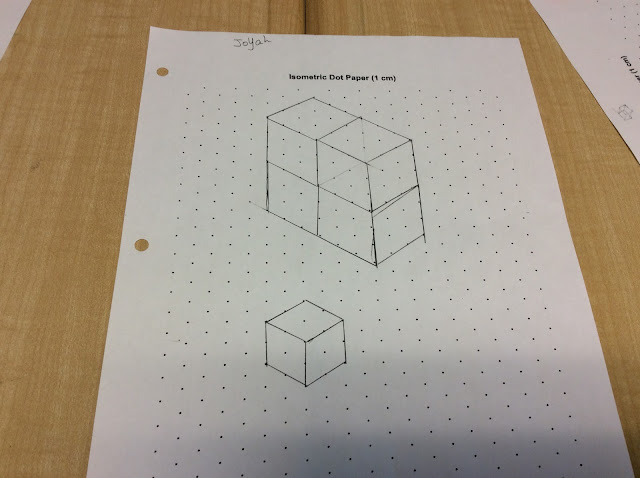
Some of my kiddies figured out Euler's Formula on their own! Way to go smartians!

For any polyhedron that doesn't intersect itself, the number of faces, plus the number of vertices (corner points), minus the number of edges always equal to 2.

F + V - E = 2

They also made other interesting observations. In order to calculate the number of faces, edges and vertices of prisms they noticed:

Number of sides + 2 (will figure out the number of faces)
Number of sides x 3 (number of edges)
Number of sides x 2 ( number of vertices)

For Pyramids:

Number of sides on base + 1 (tells the number of faces)
Number of sides on base  x 2 (number of edges)
Number of vertices and number of faces are the same.

Excellent observations kiddies!

Prisms, Pyramids or other 3D shape?

Yes, this is a pyramid! It's an oblique pyramid. It is a pyramid with an apex that is not aligned above the center of the base.